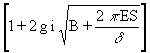¸ (1 + 2 g i)ESDEP WG 15C
STRUCTURAL SYSTEMS: MISCELLANEOUS
To outline the specific characteristics of wind loading of chimneys; to give current methods for shell buckling calculation; and to outline the importance of tolerances.
Lectures 8: Plates and Shells
Lecture 15C.1: Design of Tanks for the Storage of Oil and Water
Lecture 15C.2: Structural Design of Bins
The types of steel chimney and their associated equipment, e.g. liner, are described.
The specific aspects required in the calculation of actions are introduced, in particular, wind loads and the dynamic effects in the wind direction and perpendicular to the wind direction, and temperature loads.
Force calculations and the design of the structural shell are discussed. Specific items of design covered include buckling of cylindrical unstiffened shells, buckling of cylindrical stiffened shells, openings in cylindrical shells, and fatigue.
Fabrication and erection tolerances are introduced.
More and more chimneys are required to carry vertically and discharge to the atmosphere gaseous products of combustion, chemical waste gases, exhaust air, etc.
There are several types of steel chimneys
This lecture covers only self-supporting chimneys. The principles of calculation which are given here would have to be modified for application to other types of chimney.
There are also several types of self-supporting chimney
In double-skin or multi-flue chimneys, it is recommended that the liners are allowed to deform independently from the structural shell. If they are not independent the effects of structural deformations of the structural shell on the behaviour of the liners should be studied.
The mechanical properties and the chemical composition of structural steels should comply with the European Standard EN 10025 [1].
For high temperatures, the yield stress and the Young's modulus of steel are modified as shown in Section 2.5.
In order to limit the corrosion allowances (see Section 2.6) stainless or alloy steels should be used. Ordinary stainless steels have poor corrosion resistance in the presence of condensing sulphuric or other acids and are therefore not recommended in chimneys burning fuels containing sulphur under conditions of medium or high chemical load. They are acceptable when steel temperature is less than 65°C and acid concentration is less than 5%.
Where it is not possible to avoid high chemical loads, the use of high nickel alloy steels is a solution.
The permanent load includes the weight of all permanent parts, i.e. shell, fittings, linings, flues, and insulation and any permanent equipment.
In many cases, it is necessary to consider the carry over of ash or dust. Ash and dust may adhere to the interior surface of the structural shell or liner and cause an additional load.
The calculation of the wind actions is described according to the Model Code for Steel Chimneys edited by CIC.IND [2].
The basic wind speed, corresponding to the chimney site, is defined as the mean hourly speed at 10m above ground level in open country which occurs once every 50 years. Although steel chimneys are normally replaced after a shorter period than 50 years, the basic recurrence period is taken as 50 years and the factor of safety is determined according to a design life period (20 years for instance).
The determination of the factor of safety according to a design life period is not discussed further in the present lecture.
The design wind speed at level z above ground is obtained from the basic wind speed Vb multiplied by three factors:
V (z) = Vb k(z) kt ki... (m/s)
where
* k(z) is the height factor: k(z) = max [1 , (z/10)a]
a is equal to 0,14 if the chimney is erected in open terrain or projects well above the surrounding buildings.
* kt is the topographical factor:
kt is generally fixed by the contract. The following method for the determination of kt is valid for situations in which the chimney (height h above its foundation) is erected on a hill or escarpment which is described by:
yu : the upwind slope ³ 0,05
yd : the downwind slope ³ 0,05
U : the horizontal length of upwind slope
Then kt = 1 + 1,2 yE [1 - x/UE - h/UE] ≤ 1
where:
x is the distance of the chimney from the crest of the escarpment
yE = yu and UE = U if yu < 0,3
yE = 0,3 and 3,3 UE = U yu if yu > 0,3
* ki is the interference factor:
- if the height of the interferance object is less than half the chimney height: ki = 1
- if the interference object is an almost cylindrical structure:
ki = 1,25 - a (0,15/9d') for 6d £ a £ 15 d
ki = 1 for a > 15 d
where
a is the distance of chimney from the interference object
d' is the diameter of the interference object
- if a £ 6 d, ki should be determined by a wind tunnel test.
The mean hourly wind load per unit length of shell is:
Wm (z) = ra [V(z)]2 CD d (z) / 2 ... (N/m)
where r is the density of air: ra = 1,25 - (h1/8000) .. (kg/m3)
h1 is the altitude of the chimney site (m)
d (z) is the outside diameter of the chimney at height z (m)
CD is the shape factor which depends on Reynolds number Re:
Re = 6,9.104 V(z) d(z)
CD = 1,2 if Re £ 3.105
CD = 1,2 - 1,36 (log Re - 5,48) if 3.105 £ Re £ 7.105
CD = 0,7 if Re ³ 7.105
For chimneys with vanes (see Section 2.3.7), CD = 1,4 applied to the outer diameter of the chimney in the vaned part and not to the outer dimension of the vanes.
For attachments (such as ladders), the area presented to the wind is factored by 1,2 for circular members and by 2 for other shapes.
W (z) = Wm (z) G ... (N/m)
where
G is the gust factor which represents the influence of the fluctuating part of wind actions.
G = 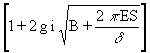¸ (1 + 2 g i)
g is the peak factor = 
with u T = 
i is the turbulence intensity = 0,311 - 0,089 log h ... (h in m)
B is the background turbulence = [1 + (h/265)0,63]-0,88
E is the energy density spectrum = 
S is the size reduction factor = [1 + 5,78 (f1/Vb)1,14 x h0,98]-0,88
f1 is the natural frequency in s-1 of the chimney oscillating in its first mode; care must be taken to include the stiffness of the foundation in the calculation of f1.
d
is the damping expressed as a fraction of critical dampingif all connections are executed by welding or prestressed bolts:
d = 
if connections are executed by ordinary bolts:
d = 
if a lining is continuously attached to the shell:
d = 
where
V is the design wind speed at the top of the chimney
t is the thickness of the wall in the top third (equivalent thickness in the case of lined chimneys calculated as the total mass per square metre divided by 7850 kg/m3
Forces due to vortex shedding cause a response of the chimney perpendicular to the wind direction. Important amplitudes occur when the shedding frequency coincides with a structural frequency.
Vortex shedding occurs at the critical wind speed:
Vcr = f1 d/St
where
St is the Strouhal number, equal to 0,2 if there is no interference object nearer to the chimney than 15 times its diameter.
If there is a cylindrical interference object - diameter d' - at a distance a which is smaller than 15 d from the chimney, the Strouhal number decreases to:
St » 0,1 + 0,1 
If a is smaller than 6d, wind tunnel tests are necessary.
Vortex shedding can be neglected if the critical wind speed exceeds 1,2 times the maximum design speed at the top of the chimney.
If not, the amplitude y of the movement of the top of the chimney in the cross-wind direction is calculated as follows:
= F (K)
where:
d1 is the diameter of shell averaged over the top third of its height
K = 
with mo =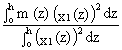 
where
x1 (z) is the mode shape of the first resonance frequency.
d
is the damping ratio calculated from Section 2.3.4 with V=0Table 1 Values of 
= F (k)
|
K |
0,47 |
0,70 |
0,86 |
1,27 |
|||
|
Re < 6.105 |
-0,23 K + 0,565 |
- 1,33 K + 1,723 |
0,032 |
||||
|
6.105 £ Re £ 3.106 |
-0,28 K + 0,565 |
- 1,465 K + 1,585 |
0,032 |
||||
|
3.106 < Re |
-0,24 K + 0,285 |
-0,609 K + 0,458 |
0,032 |
||||
Bending moments are calculated from the first mode shape normed on y at the top of the chimney
The uneven wind pressure distribution around the circumference of a circular cylinder causes bending moments in vertical cross-sections of the shell:
Mmax = 0,09 W5 sec (z) d2 (z) ... (N)
where
W5 sec is the pressure averaged over 5 seconds:
W5 sec (z) = ra (1,4 Vb)2 
/
2 ... (N/m2)
To avoid ovalling vibrations caused by vortex excitation resulting in amplified bending moments, the use of stiffening rings is suggested.
The maximum distance between rings equals: 
The minimum second moment of area about a vertical axis is:
0,4´ 10-5 ´ 103,5 ´ t0,5
Cross-wind vibrations usually can be reduced by aerodynamic stabilizers. The useful effect of three helical vanes has been proved; the radial width of the vanes is 10% of the diameter, the pitch of vanes is 5d and the vanes are fitted over the upper third of the height of the chimney.
The extra wind drag must be considered.
If there is no object causing interference to the wind flow within an effective distance, cross-wind actions on a fitted chimney can be neglected. In other cases, e.g. where there are nearby chimneys, the fitting of aerodynamical stabilizers remains beneficial but cannot be calculated.
The earthquake stress on a steel chimney is usually less than the wind loading stress. Normal steel chimneys can generally resist earthquake with an intensity of up to Mercalli scale 10 without serious damage.
However, in cases where a heavy mass is fitted at the top of the chimney, a special investigation is necessary.
The main effect of high temperature in self-supporting chimneys is the modification of the mechanical properties of the steel:
(1) Young's modulus: for temperature T between 100 and 400°C
ET = E (1 + (15,9 10-5) T - (34,5 10-7) T2 + (11,8 10-9) T3 - (17,2 10-12) T4)
(2) Yield stress: for temperature T between 100 and 400°C
fy.T = fy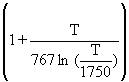 
When a chimney is restrained from adopting a distorted shape under differential expansion, bending stresses are introduced in the shell.
Stresses are high when a single unlined chimney carries gases from several sources at different temperature or when a single side entry source introduces gases at high temperature. In addition, the resulting differential steel temperature introduces secondary thermal stresses. Typically restraint occurs in bracketed, stayed or guyed chimneys.
For bare steel chimneys, the metal temperature can be assumed to be midway between ambient air temperature and that of the flue gas over the range of flue gas velocity 5 - 15m/s.
The degree of chemical load is in relation with the number of operating hours (n) when the temperature of the surface in contact with flue gases is below the estimated acid dew point + 20°C:
(1) For a S03 content of 15 ppm, the chemical load is low if n £ 25, medium if 25 < n £ 100, and high if n > 100
(2) For a different S03 contents, limit values of n vary inversely with S03 content.
(3) Where chlorides or fluorides are present in the flue gas, chemical load is high if n ³ 25.
Depending on the degree of chemical load, thickness of the steel shell is increased by an internal corrosion allowance as follows:
|
Temperature of metal in contact with flue gas |
Chemical load |
Design life |
|
|
10 yrs |
20 yrs |
||
|
< 65°C |
high |
not recommended |
|
|
65°C - 345°C
|
low |
1mm |
1mm |
|
medium |
2mm |
4mm |
|
|
high |
not recommended |
||
|
> 345°C |
low |
1mm |
1mm |
The thickness of steel shell is, in the same way, increased by an external corrosion allowance as follows:
|
Exposure |
Design life |
|
|
10 yrs |
20 yrs |
|
|
painted carbon steel |
nil |
1mm |
|
carbon steel protected by insulation/cladding |
nil |
1.5 mm |
|
unprotected carbon steel |
1,5mm |
3mm |
|
unprotected "corten" or similar steel |
1mm |
2mm |
|
unprotected stainless steel |
nil |
nil |
During design of the structural shell the following verifications are required:
(1) a check of the load resistance, in order to show that the stresses resulting from the service loads multiplied by the partial factors gi do not exceed the resistance of the shell (strength and stability).
(2) a check of serviceability in order to show that the deformations of the shell under service loads is acceptable.
(3) a fatigue check which is carried out if the loads due to vortex shedding cannot be neglected.
The main equations for checking the resistance are:
(1) sN* + sM* £ sK/gm
where sN* is the normal stress due to simultaneous factored loads
sM* is the bending stress due to simultaneous factored loads (if vortex shedding effects cannot be neglected). It results from the combination of bending moments in two directions).
gm is the partial safety factor for steel: gm = 1,1
sK is the critical buckling stress, where
sK = (1 - 0,4123 l1,2) fy when l £ Ö2
sK = 0,75 fy /l2 when l > Ö2
fy is the yield stress of steel at design temperature
l = (fy/a scr)0,5
scr = 0,605 Et/r
E is the Young's modulus of steel at design temperature
t is the corroded shell thickness
r is the radius of shell for the section considered.
a = 
aN and aM are in relation with maximal imperfection Wmax of the shell (see Section 5)
If Wmax is less than 0,04 
, then:
aN =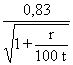 
for r/t £ 212
aN =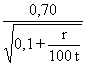 
for r/t £ 212
aM = 0,189 + 0,811 aN
If Wmax is between 0,04 and 0,08 , then:
a calculated as above is multiplied by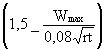 
(2) 
< 
where sx* = sN* + sM*
sy* is the factored bending stress due to ovalling
T* is the factored shear stress.
Second order effects are taken into consideration if the value of h 
exceeds 0,6,
where h is the height of the chimney
N is the total axial load
EI is the stiffness of the cross-section at the base of the chimney.
The deflection at the top of the chimney is limited to 
.
The deflection due to vortex shedding should not be greater than 0,3 d from centreline.
The fatigue check ascertains that the loading due to vortex shedding will not result in initiation and propagation of cracks in the steel material.
It shows that the difference Ds = |smax - smin| of nominal stresses in the construction detail considered does not exceed the fatigue strength DsR divided by the factor of safety gR:
Ds £ 
g
R = 1,10 if the steel temperature is less than 200°C;g
R = 1,32 if temperature is more than 200°C.Ds
R is given by the following equation:log DsR = -
log N + C
where N is the number of stress cycles
N = 0,4 x 108 x A2 e-A² f1
where A = 3,5 
Vcr is the critical wind speed for vortex shedding
Vh is the design wind-speed at the top of the chimney
m = 3 for N £ 5.107 and m = 5 for N > 5.107
C = log [cat] + 2,1
where [cat] is the category number of the constructional detail for which the fatigue check is required, see Eurocode 3 [3].
These connections are generally welded "shell on shell".
In some cases, they are bolted connections with external flanges.
For welded connections, the design should be such that the fatigue strength is as high as possible. The welding process is designed in order to limit shell deformation.
For bolted connections, the design of the bolts and the flanges takes account of the prying effect in the tension zone.
At the base, the cylindrical shell is welded onto a ring which is connected to the reinforced concrete foundation by anchor bolts.
The best design is a ring with bolts on the inner and the outer sides of the shell. The forces in the anchor are calculated by considering a mixed section, compressed concrete and extended bolts. The axis of this section is determined by calculation.
Where the ring is an external one, the prying effect is considered to amplify the bolt forces.
Where large apertures are cut in the shell plates, as for inlets or inspection panels, a structural analysis is made.
The shell is reinforced around the aperture by a frame which consists of U sections having their two flanges welded on the shell.
The profile is chosen by calculating the frame for the wind blowing parallel to the aperture on the one hand, and perpendicularly to the aperture on the other hand.
The importance of the value W of the shell imperfections is indicated in Section 3.1. The load-carrying resistance of the shell depends on Wmax.
After the erection of a chimney, shell imperfections must be measured systematically. A ruler should be used which has a length of 4 , where r is the radius of shell and t its thickness. W is the distance between the shell and the ruler. The ruler which is placed vertically is straight. The ruler which is placed horizontally is curved at the nominal radius of the shell.
[1] BS EN 10025, 1990, Hot Rolled Products of Non-Alloy Structural Steels and their Technical Delivery Conditions, British Standards Institution, London.
[2] Model Code for Steel Chimneys
CIC.IND (Comit International des Chemines Industrielles).
[3] Eurocode 3: "Design of Steel Structures": ENV 1993-1-1: Part 1.1: General Rules and Rules for Buildings, CEN, 1992.