Previous | Next | Contents

Lecture 12.7: Reliability Analysis and

Safety Factors Applied to Fatigue

Design

OBJECTIVE

To introduce the main concepts and derivation regarding both the statistical evaluation of the fatigue strength of structural details and the determination of partial safety coefficients on which are founded the fatigue assessment rules in Eurocode 3 [1].

PREREQUISITES

None.

RELATED LECTURES:

Lecture 12.8: Basic Fatigue Design Concepts in Eurocode 3

SUMMARY

This lecture presents an overview of the statistical analysis procedure applied to fatigue test data of a particular detail in order to derive the most appropriate S-N curve. Special attention is given to the definition of the partial safety factors which are in Eurocode 3 for fatigue design assessment [1]. The use of a proper fatigue damage-tolerant level is discussed which is based on sufficient residual strength and stiffness in remaining members between inspection intervals until the fatigue crack can be detected and repaired.

1. INTRODUCTION

Fatigue failure may arise in many engineering structures submitted to repeated loadings. Many failures occurring in structures are due to the process of fatigue crack propagation. Fracture may be in encountered in many civil engineering structures such as bridges, cranes, gantry girders, offshore or marine structures, transmission towers, chimneys, ski lifts, etc. The safe-life prediction of structures subjected to fatigue loading is recognised as being a very difficult problem. The stresses in any civil engineering structure are often caused by random loading; the properties of the materials, and the fabrication conditions for the structure may also vary in a random manner. The integrated influence of all these variables yields a wide scatter in life prediction.

As a result, in spite of the progress made in the understanding of the fatigue mechanism, and of the conservatism introduced in design against fatigue failure, design rules are still based on a "damage-tolerant approach" which is more or less substantiated by reliability analyses.

2. STATISTICAL ANALYSIS OF S-N CURVES

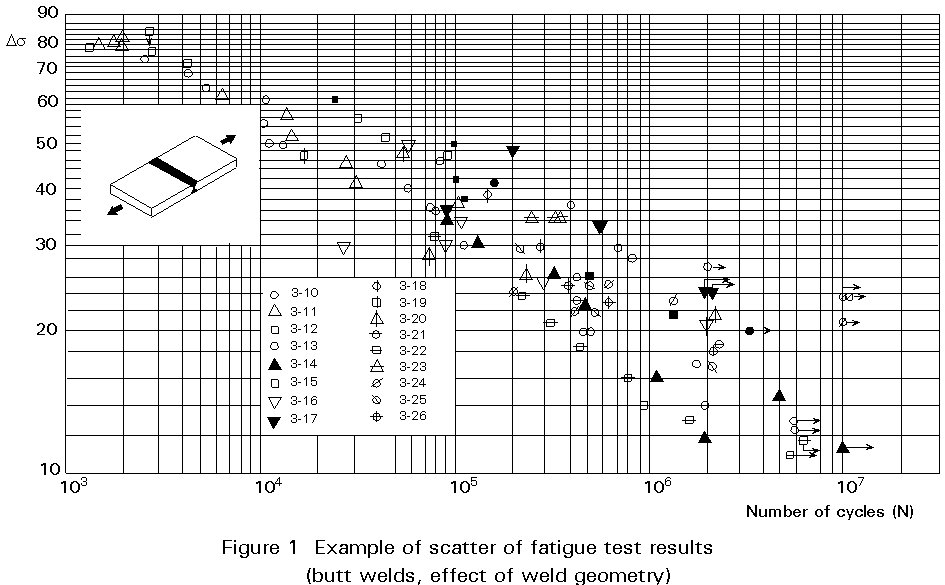
The S-N curves are evaluated from a series of fatigue tests performed on specimens which comprise the structural detail for which assessment of the fatigue strength is required. When the fatigue test results are plotted on a log-log scale, i.e. log (stress range, Ds ) versus log (number of cycles to failure) a considerable statistical scatter exists in the fatigue data as illustrated in Figure 1.

Instead of taking a lower bound limit approach, a statistical evaluation of the fatigue test results is performed. Assuming a linear relationship between log Ds and log N the following equation may be written:

log N = log A - m . log Ds (1.1)

Let

y = log N; x = log Ds ; a = log A; b = - m (1.2)

where log is the logarithm to base 10.

The first step of the statistical analysis is to apply a technique of standard linear regression analysis of the data to find estimates of a and b, denoted and   respectively. Each pair of fatigue data, y_i = log N_i and x_i = log Ds_i, should satisfy the relation:

y_i = + . x_i + e_i (1.3)

where e_i is called the residual.

The estimates and are determined so that the sum of the squares of the residuals is a minimum. This condition leads to the following estimates [2].

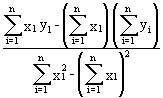= (1.4)

and

= = .                 (1.5)

where and are respectively the means of y_i and x_i, i.e.

= , = where n is the number of data points (sample size).

The second step is to find the variance of the distribution f(y_i) at a given x_i. The variance of y_i, written Var(y_i), is assumed to be constant for all values of x_i=logDs_i. The constant variance assumption is sometimes questionable, but holds true for a majority of fatigue test data. It is also usually assumed that, for any fixed value of x_i, the corresponding value of y_i forms a normal distribution.

One of the main comparison indicators which is used in relation to the classification system adopted in Eurocode 3 [1] is the characteristic strength at two million cycles, written x_c = log Ds_c. Let y_c = log N_c be the random variable corresponding to a given value x_c.

The sampling distribution of y_c may be obtained from the following estimation [2]:

_c = + .x_c (1.6)

and

(1.7)

where Var (y_c/x_c) is the variance of y_c given that x is equal to x_c.

(1.8)

where

(1.9)

A 95% confidence interval estimate for y_c is given by:

(10)

where t₉₅ is the 95% percentile of the Student's distribution with n-2 degrees of freedom [2]. Thus there is confidence that 95% of the y_c Student's t population will have values above y_ck.

Very often in fatigue test analysis the sample size is small (n£ 30) and the value of the estimation of the variance of y_c as defined by Equation (1.7) fluctuates considerably from sample to sample. To take this fact into account, the distribution of f(y_i) for sample size n £ 30 has been assumed to follow a Student's t distribution.

Knowing y_ck from Equation (1.10), the characteristic strength at two million cycles may be calculated from Equation (1.1). Finally the "one-sided" confidence estimate is performed for a particular point referred to as the characteristic strength at two million cycles.

The characteristic S-N curve is given by the following equation:

log N_k  = (log A - t₉₅s_ycxc) - m.log Ds_k     (1.11)

and the characteristic strength at two million cycles may be calculated from:

log Ds_kc = [(log A - t₉₅s_ycxc) - log (2 × 10⁶)]/m    (1.12)

Therefore

Ds_kc =10^logDskc            (1.13)

Assume a given detail has been tested under constant amplitude fatigue loading. The fatigue test data is reported in the following Table. No failure was observed for the first five specimens for a number of cycles over two million. The characteristic fatigue strength curve corresponding to the given detail is determined as follows:

Stress Range Ds (N/mm²)	Number of Cycles N (x 1000)	Failure Identification
74 74 108 108 108 108 108 139 139 202 202 202 265 265 265	Over 2000 id id id id 1077 800 597 537 204 188 107 79 70 42	not failed not failed not failed not failed not failed failed id id id id id id id id id

Table Fatigue Test Data

First Step: Perform the linear regression analysis:

The evaluation of a and b according to Equations (1.4) and (1.5) may be obtained from the following numerical Table which includes only the tests that have resulted in a fatigue failure.

b =

a =

therefore, the linear regression equation is given by:

log N = 12,334 - 3,102 log Ds (1.11)

for N_c = 2000 000 cycles, from (1.11):

log Ds_c = 1,945 and Ds_c = 88,05 N/mm²

Second Step: To find the variance of y = log N

from the calculation table above.

and the variance:

The standard deviation is then

s_yc/xc = 0,151

The Student's t₉₅ value with n - 2 = 8 degrees of freedom can be obtained from a statistical table of the t distribution [3]:

t₉₅ = 1,860

The characteristic strength at two million cycles is then:

log Ds_kc =

Therefore:

Ds_kc = 10^1,854 = 71,5

In conclusion, referring to the basic S-N curves in Eurocode 3 (Fig. 9.6.1 in Eurocode 3), the detail analysed under the preceding statistical evaluation may be classified under category 71, Figure 2.

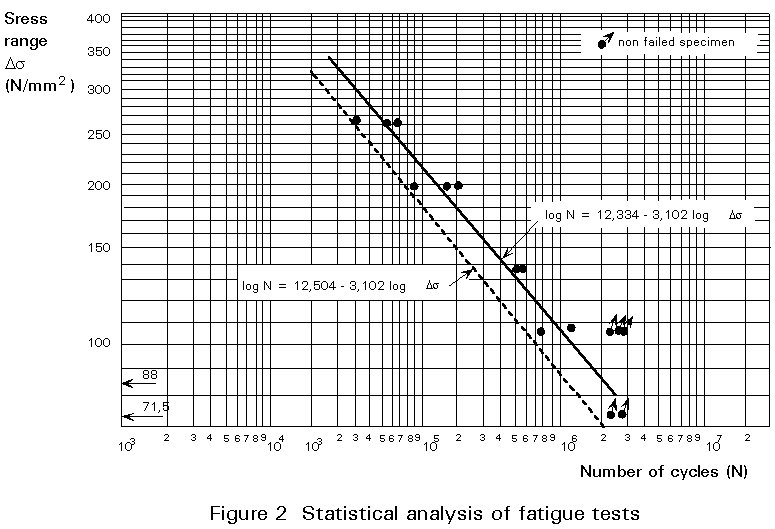
The standard deviations vary from test to test and from the type of detail studied. In general, the higher the stress concentration factor, the lower the standard deviation of the fatigue test results. The following table gives some indication of the standard deviations which were obtained when performing the statistical analysis of various types of detail category. When mixing fatigue test results from various sources, the standard deviation tends to increase and care should always be exercised to minimise problems arising from inhomogeneity of data, Figure 3. These values of the standard deviation of the number of cycles given in the table are somewhat different to the values appearing in Annex C of reference [4] due to the fact that more complete sources of fatigue data where analysed when reviewing the classification for Eurocode 3 [5].

3. SAFETY CONCEPT AND PARTIAL SAFETY COEFFICIENTS

A structural element submitted to fatigue loading is subjected to several uncertainties. The variability of the parameters governing the fatigue life of a structural element, i.e. fatigue loading and fatigue resistance are largely unknown. A level II reliability model has been implemented for the derivation of recommended partial safety factors in relation to the following fatigue strength assessment equation:

g_f Ds_s = Ds_R/g_M          (3.1)

where

Ds_s is the equivalent constant applied stress range which, for the given number of cycles, leads to the same cumulative damage as the design spectrum.

Ds_R is the fatigue strength as given by the S-N curve of the relevant detail category.

g_f and g_M are the partial safety factors applied respectively to the spectrum loading and to the resistance.

3.1 Derivation of Partial Safety Factors

It will be assumed that log Ds_s and log Ds_R are both random variables following a normal distribution law. Therefore the random variables Ds_s and Ds_R are said to follow a log-normal distribution.

The fatigue limit state function may be written as:

g = log Ds_R - log Ds_s (3.2)

Introducing the normalised basic variables u and v as:

u = {log Ds_R - } / S_logDsR   (3.3)

v = {log Ds_S - } / S_logDsS  

 is the mean value and S_logDsR is the standard deviation of the variable log Ds_R.

The limit state function, Equation (3.2), re-written with the normalised basic variables becomes:

g(u,v) = S_logDsR.u - S_logDsS.v + - (3.4)

Having assumed that the random variables log Ds_S and log Ds_R are normally distributed, then the safety index b is related to the probability of failure P as:

P = F(-b) (3.5)

in which F(-b) is the standardised normal distribution function and b is given by (it is assumed for the sake of simplicity that the variables u and v are uncorrelated):

b = { - } / Ö(S²_logDsR + S²_logDsS)     (3.6)

It is recalled that b can be geometrically interpreted as the shortest distance from the origin to the failure surface in the standard normal space, Figure 4. 

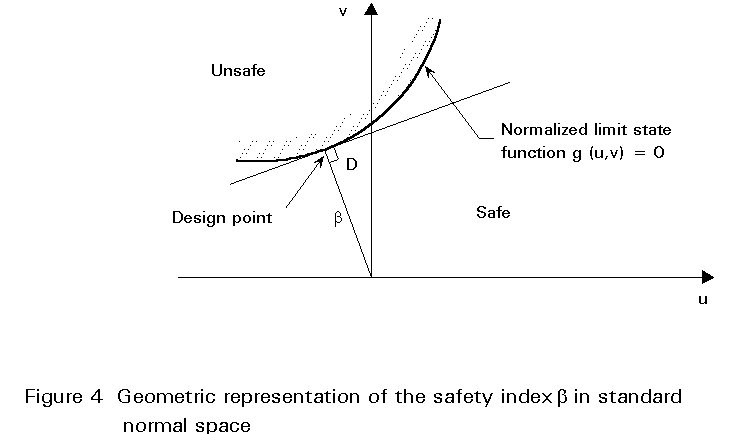
The coordinates of the design point D represent the values of u and v with the highest probability of failure. These coordinates are expressed by:

a_u = -bS_logDsR / Ö(S²_logDsR + S²_logDsS)          (3.7) 

a_v = b S_logDsS / Ö(S²_logDsR + S²_logDsS)

Rearranging Equation (3.7) in terms of the basic variables log Ds_S and log Ds_R gives:

a_R = - bS²_logDsR / Ö( S²_logDsR + S²_logDsS)                 (3.8)

a_s = + b S²_logDsS / Ö( S²_logDsR + S²_logDsS)

The characteristic values of the random variables (log Ds_Rk and log Ds_sk) are then expressed by:

log Ds_Rk = (log Ds_R)_k = (1 - k_R C_R) (3.9)

log Ds_sk = (log Ds_s)_k = (1 + k_s C_s)

where C_s and C_R are respectively the coefficients of variation of log Ds_s and log Ds_R (i.e. the ratio of standard deviation over the mean value).

To determine the partial safety factors in a semi-probabilistic format (level I reliability format), which corresponds to the same degree of safety (represented by the safety index b) as a level II reliability format, one has to utilize the Equations (3.8) and (3.9). Assuming:

m = S_{log DsR}/S_{log DsR}

then the partial factors can be obtained from the following relations:

log g_M = S_{log DsR} b /[Ö(1+ m²)] - k_R        (3.10)

log g_f = mS_{log Dss} bm /[Ö(1+ m²)] - k_s

For a defined safety index b the partial safety coefficients may be calculated from Equations (3.10), knowing the standard deviations of the resistance and the loading (S_{log DsR} and S_{log Dss}) and the coefficients k_R and k_S related to the definition of the characteristic values adopted for (log Ds_R)_k and (log Ds_s)_k.

• As shown in Section 2, there is sufficient experimental data to determine adequate values of the standard deviation of the resistance S_{log DsR}.
• On the other hand there is little information concerning the variation of the fatigue loading. The standard deviation of the fatigue loading, (S_{log Dss}), must be evaluated or estimated and depends very much upon the type of traffic load (railways, roadway or highway). Since the distribution of Ds_s is lognormal, then the standard deviation of log Ds_s may be expressed in terms of the coefficient of variation of the loading as:

S_{log Dss} = log e Ö[ln(1 + V_s²)] (3.11)

with by definition:

V_s = S_Dss /

where e is the Euler's number and ln is the Naperian logarithm.

• The formal difference in the ECCS Fatigue Recommendations is due to the fact that the characteristic value of the loading has been introduced in a probabilistic format, which is not the case in the ECCS Recommendations where the fatigue loading is defined by its mean value.
• The partial safety coefficients depend upon the required safety index. From the viewpoint of the fatigue reliability assessment, there are many "critical" structural components or structural details which must be considered in any civil engineering structure. However, it must be recognised that failure of a particular structural component in a structure does not necessarily imply a complete failure of the structure. A distinction must be made between the notion of "fail-safe" and "non fail-safe" structural components. This notional concept is exemplified in Figure 5: It has to be understood that in a "fail-safe" assembly, the result of a normal failure is a loss of rigidity, but the structure retains its integrity. Nevertheless, it is necessary to assess a safe life capability of structural components whose fracture may potentially give rise to a catastrophic failure. Safe-life design should be required especially for structural components or details for which inspection is difficult, or cannot be properly carried out.
• The periodic inspection and maintenance of the construction in conjunction with the variations in accessibility of the structural detail for inspection or repair must be taken into consideration when evaluating the risk and assessing the proper safety indices.
• In the same structure, there are components which may be classified as "fail-safe" and others as "non fail-safe" from the viewpoint of failure consequences.

It must be understood that the safety indices which were proposed (see Table 1) in Eurocode 3 (Chapter 9) are mainly based on an engineering judgement of what may be called a potential risk of acceptance of losses and damages. It is the responsibility of each concerned authority to make the decision on the proper choice of these values on the basis of a realistic risk assessment.

	"Fail-Safe" structural detail	"Non fail-safe" structural detail
Periodic inspection and maintenance. Accessible joint detail.	b = 2	b = 3
Periodic inspection and maintenance. Poor accessibility.	b = 2,5	b = 3,5

Table 1 Recommended values of safety indices

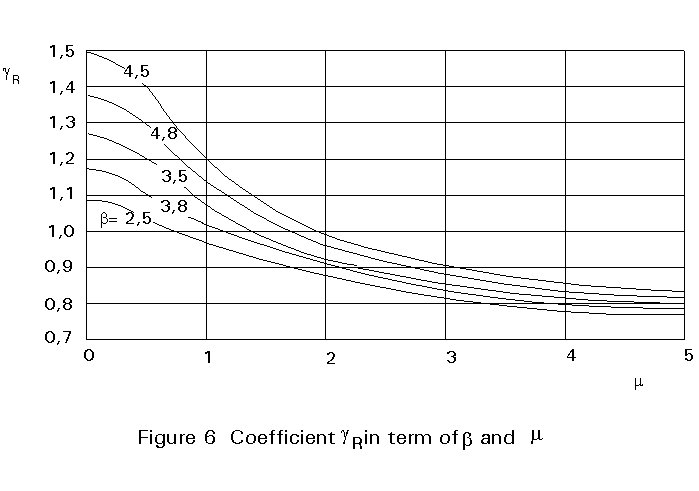
Figure 6 gives the partial safety coefficient g_R in terms of b and m. These curves have been drawn for k_R=2 and k_S=1,645 and for s_logDsR=0,07 (which corresponds to s_logN=0,210.

In Chapter 9 of Eurocode 3 [1] values of the product g_f.g_M have been proposed (Table 2) based on the values of safety indices given in Table 1. There is little information concerning fatigue loadings and if the partial safety factor g_f is known, then the partial safety factor g_M related to the strength must be adjusted.

	"Fail-safe" structural detail	"Non fail-safe" structural detail
Periodic inspection and maintenance. Accessible joint detail.	g = 1,00	g = 1,25
Periodic inspection and maintenance. Poor accessibility.	g = 1,15	g = 1,35

Table 2 Recommended values of the product g = g_f.g_M

Discontinuities play a major role in the fatigue strength, particularly for welded details. Careful consideration must be given to the weld quality since it significantly affects fatigue strength variation. Moreover, the measures that can be taken to achieve the required degree of structural reliability include not only the justification of relevant design rules and the choice of associated partial safety factors, but also an appropriate level of execution quality and proper standards for workmanship which are developed in pr EN 1090-1 [6].

4. CONCLUDING SUMMARY

• Opinions regarding the fatigue resistance of a structure vary from the extreme that the structure should be safe during the design life under any circumstances (or during damage that may be inflicted) to the view that safe-life cannot be predicted, or a reasonably economic life goal cannot be assured.
• The purpose of a code is to set a set of partial safety factors which minimise the rate of fatigue damage in service, and to take advantage of suitable inspection and proper maintenance procedures.
• In structures designed to Eurocode 3, since a completely fatigue-resistant structure is unlikely to be economically feasible, some fatigue cracks during service should be expected.
• "Fail-safe design" can best serve a useful purpose when supplemented by suitable inspection and maintenance. The design concept in Eurocode 3 may be described as follows:

(i) The structure must have an adequate life either crack-free or during which the growth rate of cracks is sufficiently low to allow detection during an inspection period.

(ii) Visual inspection of all critical areas is possible in service.

5. REFERENCES

[1] Eurocode 3: "Design of Steel Structures": ENV 1993-1-1: Part 1.1, General Rules and Rules for Buildings, CEN, 1992.

[2] Walpole E.R. and Myers R.H., Probability and statistics for engineers and scientists, MacMillan Publishing Co. Inc., New York, 2nd Ed., 1978

[3] Natrella M.G., Experimental Statistics, National Bureau of Standards Handbook 91, Issued August 1 1963. Reprinted October 1966 with corrections.

[4] Recommendations pour la vrification la fatigue des structures en acier. CECM - Comit Technique no. 6: "Fatigue". CECM no. 43, 1987, Premire dition.

[5] Background Documentation to Eurocode 3: Chapter 9 - Fatigue, Background information on fatigue design rules: Statistical Evaluation, December 1989.

[6] prEN 1090-1:1993 Execution of steel structures, Part 1: General rules and rules for buildings.

Previous | Next | Contents