ESDEP WG 4B
PROTECTION: FIRE
To make designers familiar with simple methods of calculation of fire resistance time and thickness of insulation for columns and beams (respectively steel and composite steel-concrete).
None.
Lecture 4B.1: Introduction to Fire Safety
Lecture 4B.2: Background to Thermal Analysis
Lecture 4B.3: Background to Structural (Mechanical Fire) Analysis
Lecture 4B.4: Practical Ways of Achieving Fire Resistance of Steel Structures
Calculation examples are presented for the following:
The examples use the principles and design equations presented in the preceding lectures.
EXAMPLE 1 CRITICAL TEMPERATURE OF TENSION MEMBER
|
Strength reduction of steel at elevated temperatures |
||||||
|
Temperature q |
400 |
450 |
500 |
550 |
600 |
650 |
|
Strength Reduction y(q) |
1,00 |
0,93 |
0,78 |
0,63 |
0,47 |
0,33 |
For ![]()
As the performance of a member in tension is equivalent to the basic performance of the steel, it follows that:
![]()
By linear interpolation from the above table, the critical temperature qcr = 590°C
EXAMPLE 2 CRITICAL TEMPERATURE OF BEAM
It is assumed in this example that the beam supports a concrete slab, and hence the upper flange remains cooler than the rest of the section. This benefit is taken into account by use of a load multiplier, or kappa factor, k, such that:
![]()
k
= 0,7 for a beam supporting a concrete slabUse the same degree of loading as in Example 1.
In this case, ![]()
For ![]() = 0,5, it follows that:
= 0,5, it follows that:
y
(q) = 0,7 x 0,5 = 0,35By linear interpolation of the strength reductions in Example 1, the critical temperature of the beam, qcr = 645°C.
It follows that the critical temperature of a beam supporting a concrete floor slab exceeds that of a member in tension, i.e. uniformly heated, by 55°C for the same degree of loading.
EXAMPLE 3 CRITICAL TEMPERATURE OF COLUMN
It is assumed in this example that the column is restrained against buckling. The load multiplier, k, for columns is 1,2. This value takes into account the influence of high strains in the column at failure in fire conditions.
For ![]() , as in previous examples
, as in previous examples
y
(q) = 1,2 x 0,5 = 0,6By linear interpolation of the strength reductions in Example 1, the critical temperature of the column, qcr=560°C.
It follows that the critical temperature of a column is less than that of a member in tension by 30°C for the same degree of loading.
EXAMPLE 4 FIRE PROTECTION TO STEEL BEAM
From Example 2, the critical temperature of the beam is 645°C. From Lecture 4B.2, the required thickness of fire protection (in metres) is:
d = 0,0083 li (Am/A) {t/(qcr -140)}1,3
Where
Am/A is the section factor of member (m-1)
l
i is the thermal conductivity of protection material (W/m°C)t is the fire resistance period (mins)
q
cr is the critical temperature of beam (°C)In this Example, use the following parameters:
Am/A = 200m-1 (typical of IPE beams)
l
i = 0,15W/m°C (typical of many protection materials)t = 60 mins
q
cr = 645°Cd = 0,0083 x 0,15 x 200 x {60/(645 -140)}1,3 ×10-3
= 15,6 mm (say 16 mm)
EXAMPLE 5 MOMENT RESISTANCE OF COMPOSITE BEAM
This Example follows the use of the principles of plastic analysis to calculate the moment resistance of a composite beam in fire conditions. The following properties are assumed:
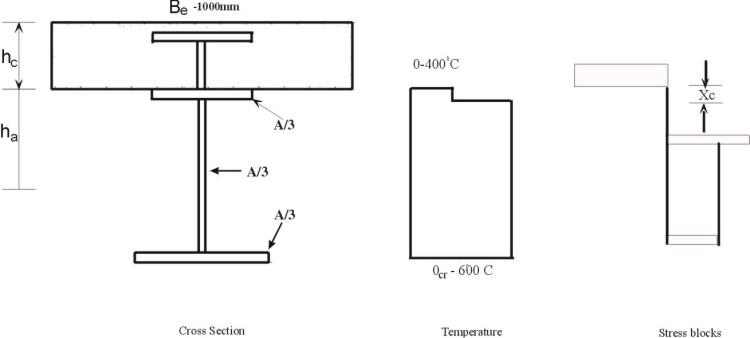
Cross-section Temperature Stress blocks
Cross-sectional area of web = area of flange
Temperature of top flange = 2/3 x temperature of web and bottom flange
Effective breadth of slab = 1000 mm
Compressive strength of concrete fc = 30 N/mm2 (Note: for short term load in fire conditions gmc = 1,0)
Yield strength of steel fy = 235 N/mm2
Critical temperature of beam qcr = 600°C (assumed)
Neutral axis depth, xc, in concrete is obtained by equating tension and compression. Hence:
![]()
y(600) = 0,47 |
} |
|
|
} from Example 1 |
y(400) = 1,00 |
} |
![]()
Moment resistance of composite section is obtained by taking moments about the mid-length of the concrete in compression:
M = ![]()
+ ![]()
M = 0,47 A fy (0,5 ha + 1,44 hc - 0,72 xc)
For the following data:
ha = 400 mm
hc = 120 mm
A = 1000 mm2
xc = ![]() in fire conditions
in fire conditions
M = 0,47 x 1000 x 235 x (0,5 x 400 + 1,44 x 120 - 0,72 x 5) x 10-6
= 40,8 kNm
By comparison under normal conditions, using y = 1,0, moment resistance is 74,2 kNm (note: this value may be calculated using the partial safety factors appropriate for normal conditions, as covered in the lecture on Composite Beams).
Therefore M/Mu = 40,8/74,2 = 0,58 for qcr = 600°C
But y(600) = 0,47
If y(600) = k ´ M/Mu, it follows that:
k
= 0,47/0,58 = 0,81 for composite beams (compare to 0,7 for non-composite beams)EXAMPLE 6 TIME-EQUIVALENT OF NATURAL FIRE
Refer to Lecture 4B.1. Assume that the fire compartment may be characterized by the following parameters. The time equivalent is:
Te = c w qf minutes
c = 0,10 for typical compartment properties
w = 1,5 for typical ventilation conditions
qf = 450 MJ/m2 for office buildings
Te = 0,1 x 1,5 x 450 = 67,5 minutes