
ESDEP WG 1B
STEEL CONSTRUCTION:
INTRODUCTION TO DESIGN
To provide an introduction to the sources of loads on structures and how loads can be quantified for the purpose of structural design.
Lecture 1B.2.1: Design Philosophies
Various types of loads (dead, imposed and environmental) and their classification as permanent, transient or accidental within Eurocode 1: Basis of Design and Actions on Structures, is considered. Calculations for dead loads on the basis of material densities and component sizes are explained. Means of estimating imposed loads based upon usage and the implications of change of use are discussed. Loads due to snow, temperature and seismic effects are considered briefly. The statistical treatment of wind and wave loads, and their dependence upon wind speed and wave height respectively, are described. The importance of load characteristics, other than simply their magnitude, is considered. These characteristics include fatigue, dynamic and aerodynamic effects. Simplified treatments for dynamic loads are described.
Structures are subject directly to loads from various sources. These loads are referred to as direct actions and include gravity and environmental effects, such as wind and snow. In addition deformations may be imposed on a structure, for instance due to settlement or thermal expansion. These 'loads' are indirect actions. In applying any quantitative approach to structural analysis, the magnitudes of the actions need to be identified. Furthermore, if the structure is to perform satisfactorily throughout its design life, the nature of the loads should be understood and appropriate measures taken to avoid problems of, for instance, fatigue or vibration.
The magnitude of loads cannot be determined precisely. In some cases, for instance in considering loads due to the self-weight of the structure, it might be thought that values can be calculated fairly accurately. In other cases, such as wind loads, it is only possible to estimate likely levels of load. The estimate can be based on observation of previous conditions and applying a probabilistic approach to predict maximum effects which might occur within the design life of the structure. (In fact, the extensive wind records which are now available mean that wind loads can often be predicted with greater accuracy than self-weight). Loads associated with the use of the structure can only be estimated based on the nature of usage. Insufficient data is available in most cases for a fully statistical approach and nominal values are therefore assigned. In addition, problems of change of use and fashion can occur.
In analysing structures it is rare to consider all loadings acting simultaneously. This approach may be because the most severe condition for parts of the structure occurs when some other combination of load is considered. Alternatively it may be that the possibility of such a condition actually occurring is extremely small. However, the risk of coexistence of apparently unrelated loads may be greater than is first imagined. Correlations can be produced from unexpected sources or from coincidences which, although physically unconnected, are temporarily connected. For example, floor and wind loads would normally be considered as unrelated. However, in hurricane areas residents on the coast might be expected to move their ground floor contents to upper floors if a hurricane warning, with associated tidal surge, were given. This circumstance could very easily produce extreme floor loads in combination with extreme wind loads. This case may be a very special one but there are others. The risk of fire may not be considered correlated with high wind loads, yet in many parts of the world high winds are more likely in winter, which is also the period of greatest fire risk.
For these reasons it is convenient to consider loads under various categories. The categories can then be ascribed different safety factors and applied in various combinations as required. Traditionally, loadings have been classified as dead, superimposed and environmental loads. These classes include a wide range of gravity effects, seismic action, pressures due to retained material or liquids, temperature induced movement, and, for marine structures, water movement. The Eurocodes on actions and steelwork design [1, 2] classify loads and other actions as permanent, variable and accidental. These classes of action will be considered in more detail in the following Sections.
In limit state design, characteristic values of actions are used as the basis of all calculations. They are values which statistically have only a small probability of being exceeded during the life of the structure. To provide a margin of safety, particularly against collapse, partial safety factors are applied to these characteristic values to obtain design quantities. In principle, different partial safety factors can be applied depending on the degree of uncertainty or variability of a particular type of action. In practice, whilst this appears to be the case, the actual values of partial safety factors used incorporate significant elements of the global safety factor and do not represent a rigorous probabilistic treatment of the uncertainties of the actions.
Permanent actions, as the name implies, are always present and must be considered in all cases. They comprise what are traditionally referred to as dead loads, but may also include permanent imposed loads due, for instance, to machinery or stored material.
Dead loads are gravity loads due to the self weight of the structure and any fixtures or finishes attached to it (Figure 1). Their magnitudes can be estimated with reasonable confidence based on prescribed dimensions and a knowledge of material density. Even so, variations due to constructional tolerances and natural variations in materials, will exist. Furthermore, fixtures, fittings and finishes may be replaced or modified during the life of the structure. This possibility has been recognised in calculating loads on bridge decks, for which a separate load category of 'superimposed dead load' is included to allow for surfacing which is likely to be replaced a number of times during the life of the bridge. For this situation there is consequently a much greater potential for variability than for other dead loads.

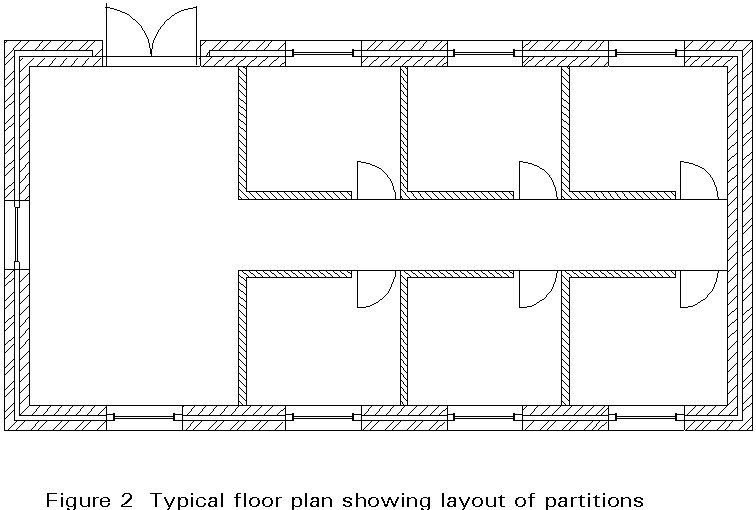
A similar condition exists within certain types of building with respect to partitions (Figure 2). Where the position of walls is predetermined their weight can simply be included as a dead load. For more speculative development, internal partitions will be the responsibility of the client and their layout is likely to change many times during the life of the building. An allowance, as an equivalent uniformly distributed load, is therefore normally made.
Schedules of densities for common building materials are listed in Eurocode 1 [1] and manufacturers of proprietary products, such as cladding, blockwork, raised floors, etc. provide information on weights. Together with specified dimensions, these data enable dead loads to be calculated. Where dead loads are not strictly evenly distributed over a plan area, such as timber floor joists located at discrete intervals, they are often represented as an equivalent uniformly distributed load for convenience in design calculations. As long as the equivalent magnitude is determined in a rational manner, any differences between this simplified approach and a more rigorous analysis taking account of the actual location of the joists will be negligible.
To determine dead loads, consider, for example, the case of a floor consisting of a 150mm thick reinforced concrete slab with 50mm lightweight screed and a 15mm plaster soffit. Details are shown in Figure 3 together with densities for each material. The total dead load per square metre of floor plan can be calculated as follows:
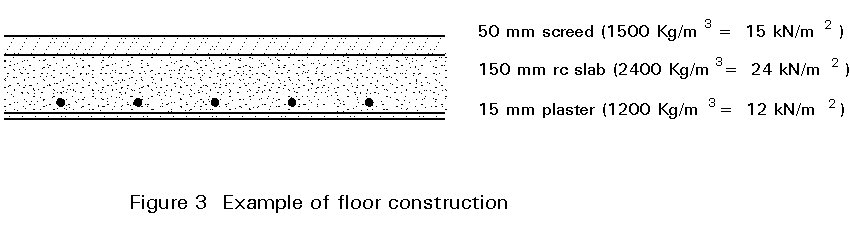
|
lightweight screed |
15 x 0,05 |
= 0,75 kN/m2 |
rc slab |
24 x 0,15 |
= 3,60 |
plaster |
12 x 0,015 |
= 0,18 |
|
total dead load |
|
= 4,53 kN/m2 |
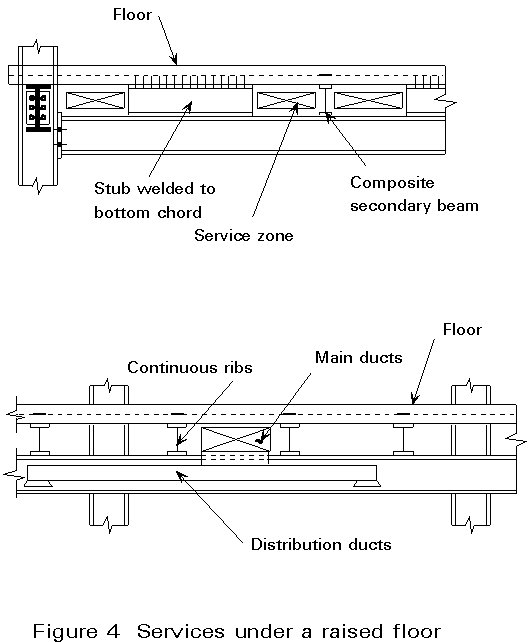
In addition an allowance would normally be made for any services or fittings (electric lighting, pipework, etc.) fitted to the underside of the slab or located within the screed or under a raised floor (Figure 4). This case is another where an equivalent uniformly distributed load is used to represent load sources distributed in an uneven manner. A value between 0,1 and 0,3 kN/m2 is normally adequate to cover such installations.
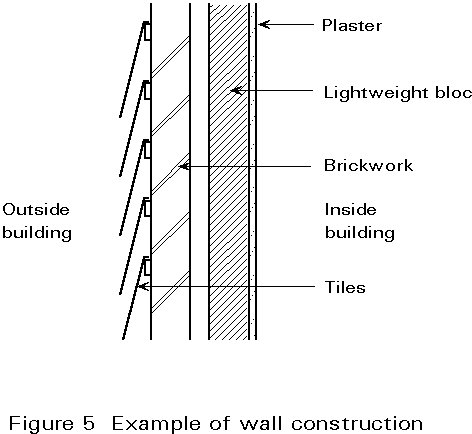
The weight of walls can be treated in a similar manner to floors by considering the various component parts and summing the weights per square metre on elevation. For example, consider a cavity wall consisting of a tile-hung brick outer leaf (100mm thick) and a plastered blockwork inner leaf (150mm thick) as shown in cross-section in Figure 5.
The total dead load is determined as follows:
|
tiles |
0,6 kN/m2 |
brickwork |
2,1 |
|
blockwork |
1,4 |
|
plaster |
0,2 |
|
total dead load of wall |
4,3 kN/m2 |
By multiplying this value by the height of the wall, the load intensity as a line load on the supporting structure can be determined.
Loads due to internal lightweight stud or blockwork partitions cannot normally be treated in such a rigorous manner since their location is often not known at the design stage and in any case may change during the life of the building. Instead an allowance is made within the assessment of imposed loads which is described under variable actions.
Variable actions comprise loads which are not always acting but may exist at various times during the normal use of the structure. They include loads due to the occupation of a building and traffic on bridges (imposed loads), snow and wind loads (environmental loads), and temperature effects (Figure 6). They do not include accidental conditions such as fire, explosion or impact.
Imposed loads - sometimes referred to as "superimposed", "super" or "live" loads - are those loads due directly to the use of the structure. For buildings, they are concerned with the occupancy by people, furniture, equipment, etc. For bridges they are due to traffic, whether pedestrian or vehicular.
Clearly these conditions will be almost constantly changing and are rather more difficult to quantify than dead loads. For buildings, the approach has therefore been to relate imposed load levels to occupancy, and to base them on observation and sensible deduction. Eurocode 1: Basis of Design and Actions on Structures [1] distinguishes between four classes of loaded floor area as follows:
The first class is further subdivided into four categories according to their specific use. They are residential (including hospital wards, hotel bedrooms etc.), public premises (such as offices, hotels, hospitals, schools, leisure centres etc.), public premises susceptible to overcrowding (including assembly halls, conference rooms, theatres, shopping areas and exhibition rooms), and public premises susceptible to overcrowding and accumulation of goods (including areas in warehouses and department stores).
The characteristic values of the imposed loads for these different categories are given in Table 1. Thus domestic residences attract a lower imposed load than office accommodation; areas of public assembly, where large numbers of people could gather at any one time, are prescribed a high superimposed load. Storage areas must be particularly carefully considered and Eurocode 1 includes details of densities for a range of stored materials. Some of these, such as steel strip, will generate high loads, but even apparently innocuous conditions, such as filing stores, can experience very high loading levels. Escape routes must be designed for relatively high imposed loads.
Although such loads are used in limit state design in a semi-probabilistic way and are referred to as characteristic values (implying a statistical basis for their derivation) little data is available. A proper statistical analysis is not therefore possible and values specified are nominal quantities. One study which was conducted into office accommodation in the UK [4] revealed a wide variation in actual load levels for similar building occupancies. In all cases the load levels measured were considerably less than the characteristic values specified for the structural design. However, this observation must be viewed with some caution since design must allow for extreme conditions, misuse and panic situations.
Note that, although imposed loading will rarely be evenly distributed, a uniform distribution of load intensity is normally assumed (Figure 7).

The nominal values of imposed load associated with different classifications of building occupancy and use represent extreme conditions. In many cases the probability of such conditions existing simultaneously throughout a building is remote. In recognition of this remote possibility some reductions in imposed load intensity may be permitted. Reduction applies particularly to columns in multi-storey buildings where it increases with the number of floors supported by a particular length of column. Typical reductions range from 10% to 30% and apply to imposed loads only. No reductions are permitted in dead load or for certain types of imposed load - notably in the case of storage areas, crane loads, and loads explicitly allowed for such as those due to machinery or due to people in public premises susceptible to overcrowding.
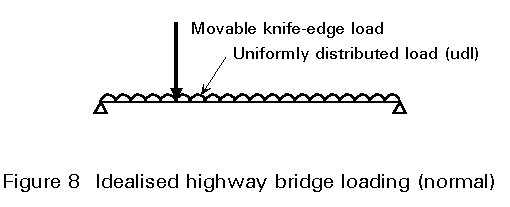
In practice a highway bridge is loaded in a very complex way by vehicles of varying sizes and groupings. In order to simplify the design process this real loading is typically simulated by two basic imposed loads - a uniformly distributed load and a knife edge load - representing an extreme condition of normal usage (Figure 8). The design is then checked for a further load arrangement representing the passage of an abnormal load. The magnitudes of all these loads are generally related to the road classification, the highway authority's requirements and the loaded length of the bridge.
For vehicular traffic within buildings, lightweight conditions (less than 16 tonnes) can be dealt with in categories such as cars, light vehicles and medium vehicles. For heavier traffic, highway loading must be considered.
Railway bridge design must take account of static loading and forces associated with the movement of vehicles. As for highway bridges, two models of loading are specified for consideration as separate load cases. They represent ordinary traffic on mainline railways and, where appropriate, abnormal heavy loads. They are expressed as static loads due to stationary vehicles and are factored to allow for dynamic effects associated with train speeds up to 300km/h. Eurocode 1 also gives guidance on the distribution of loads and their effects and specifies horizontal forces due to vehicle motion. Centrifugal forces associated with the movement around curves, lateral forces due to oscillation of vehicles (nosing) and longitudinal forces due to traction and braking are included.
Other aspects of bridge loading which need to be considered include accidental loads and the possibility of premature failure due to fatigue under traffic loading.
For buildings fitted with travelling overhead cranes, the loads due to the crane itself and the lifted load are considered separately. The self weight of the crane installation is generally readily available from the manufacturer, and the load lifted corresponds to the maximum lifting capacity of the crane. When a load is lifted from rest, there is an associated acceleration in the vertical direction. In the same way that gravity loads are equal to mass multiplied by the acceleration due to gravity, so the lifting movement causes an additional force. If the load is lifted very gently - that is with little acceleration - this force will be very small, but a sudden snatch, i.e. a rapid rate of acceleration, would result in a significant force. This force is of course in addition to the normal force due to gravity, and is generally allowed for by factoring the normal static crane loads.
Movements of the crane, both along the length and across the width of the building, are also associated with accelerations and retardations, this time in the horizontal plane. The associated horizontal forces must be taken into account in the design of the supporting structure. The magnitude of the forces will depend, as before, on the rates of acceleration. The normal procedure is to calculate the magnitudes on the basis of a proportion of the vertical wheel load.
The approach yields an equivalent static force which can be used in designing the structure for strength. However, the nature of crane loads must also be recognised. The possibility of premature failure due to fatigue under the cyclic loading conditions should be considered.
Environmental loads are clearly variable actions. For bridges and buildings the most important environmental loads are those due to snow and wind. For marine structures, particularly offshore installations such as oil platforms, loads due to water movements are often dominant. The action of waves generally represents the most severe condition. In certain geographical locations, the effects of earthquakes must be included in the structural analysis. All of these loads from environmental sources are beyond the control of man. It has therefore been recognised that a statistical approach must be adopted in order to quantify corresponding design loads.
The approach is based on the 'return period' which is a length of time to which recorded environmental data, such as wind speeds, snowfall or wave heights, is related. If records are only available over a relatively short period, data for the 'return period' may be predicted. The most severe condition on average over the return period then represents the design value. For a return period of 100 years, for example, it is referred to as the 1 in 100 year wind speed or wave height, etc. The return period normally corresponds to the design life of the structure. Clearly there is a degree of uncertainty about the process of predicting the most severe conditions likely to be encountered. Further simplifications are implicit in translating measured environmental data such as wind speeds or wave heights into loads.
Wind forces fluctuate with time but for many structures the dynamic effect is small and the wind load can be treated using normal static methods. Such structures are defined as 'rigid' and Eurocode 1 [1] provides guidance on this classification. For slender structures the dynamic effect may be significant. Such structures are classified as 'flexible' structures and their dynamic behaviour must be taken into account.
The most important parameter in quantifying wind loads is the wind speed. The basis for design is the maximum wind speed (gust) predicted for the design life of the structure. Factors which influence its magnitude are:
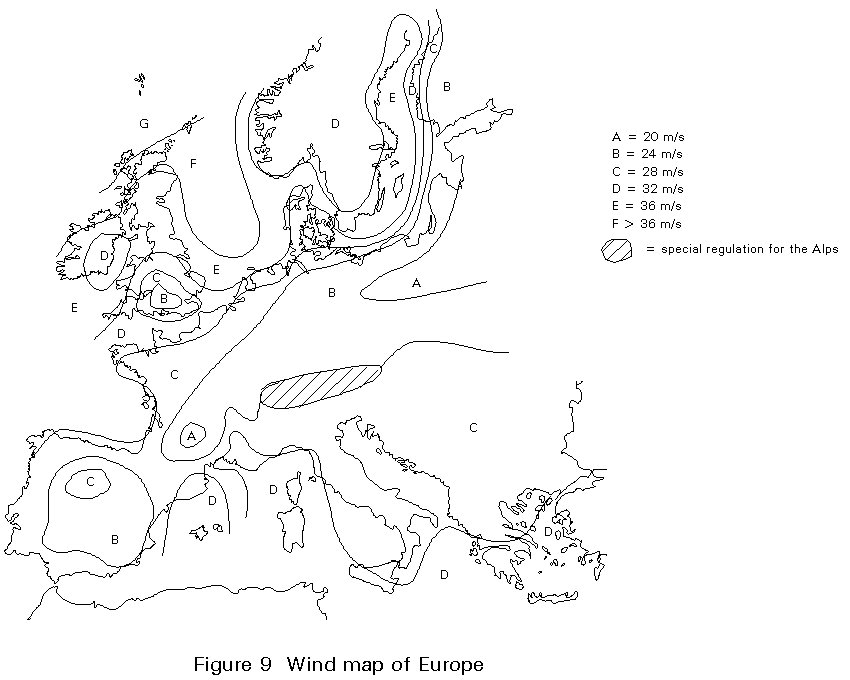
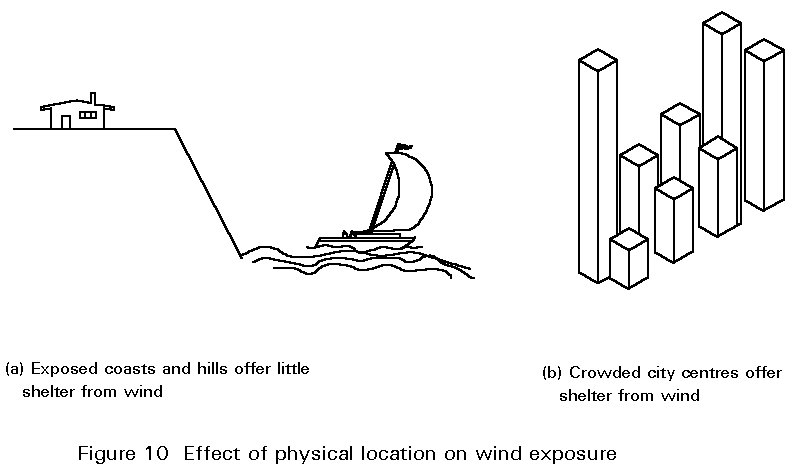

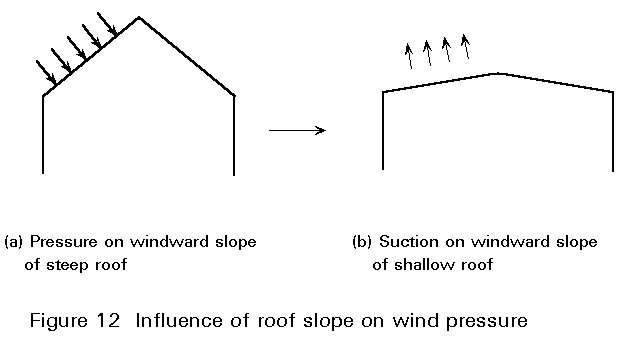
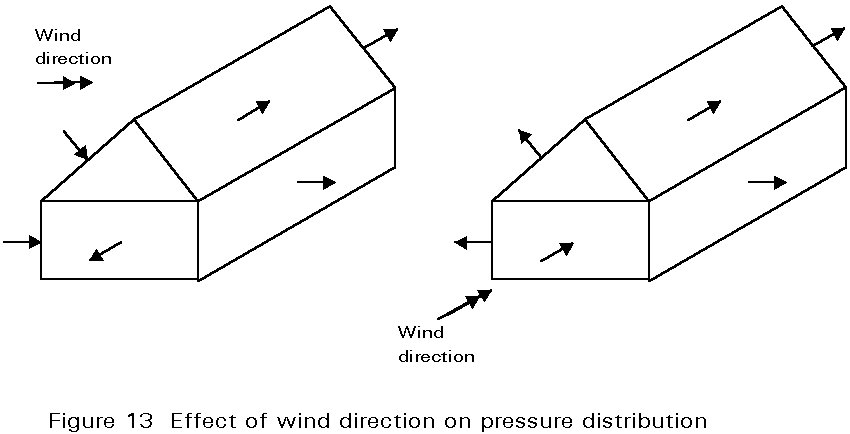
Tabulated procedures enable the above parameters to be accounted for firstly in calculating the design wind speed, and secondly in translating that wind speed into a system of forces on the structure. These equivalent static forces can then be used in the analysis and resistance design of the structure, as a whole. However, certain additional features of wind should also be taken into account:
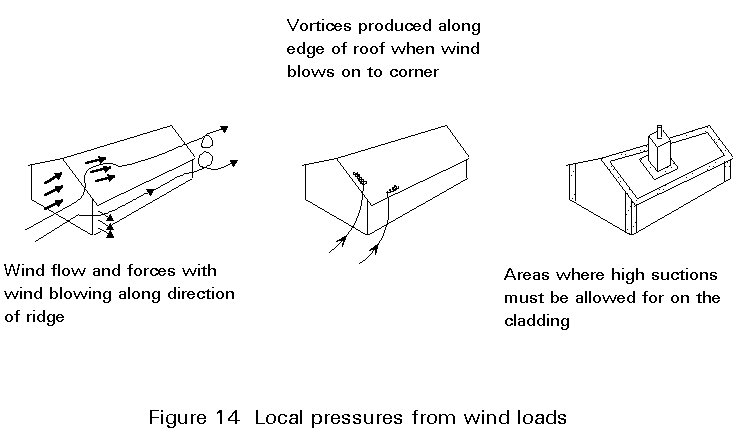
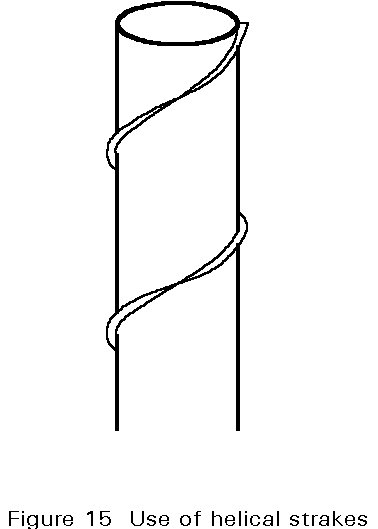
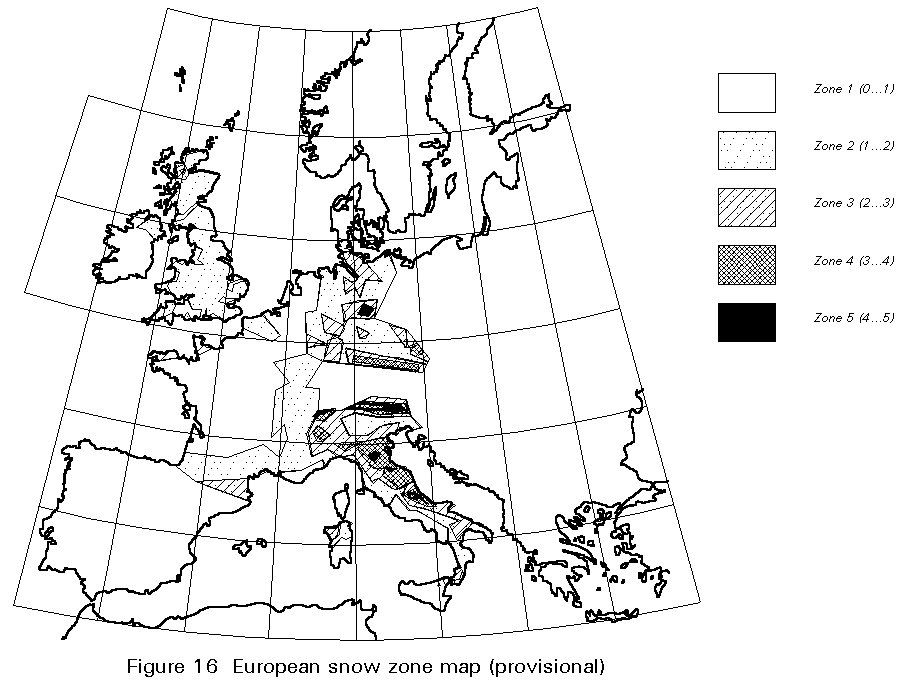
Loads due to snow have traditionally been treated by specifying a single load intensity, with possible reductions for steep roof slopes. This approach takes no account of such aspects as the increased snowfall at higher altitudes or of locally higher loads due to drifting. Cases of complete or partial collapse due to snow load are not unknown [5]. A more rational approach is to use a snow map giving basic snow load intensities for a specified altitude and return period similar to the treatment for basic wind speeds (Figure 16). Corrections for different altitudes or design life can then be applied as shown in Table 2. At present the European snow map is provisional and further work is under way to acquire more data.
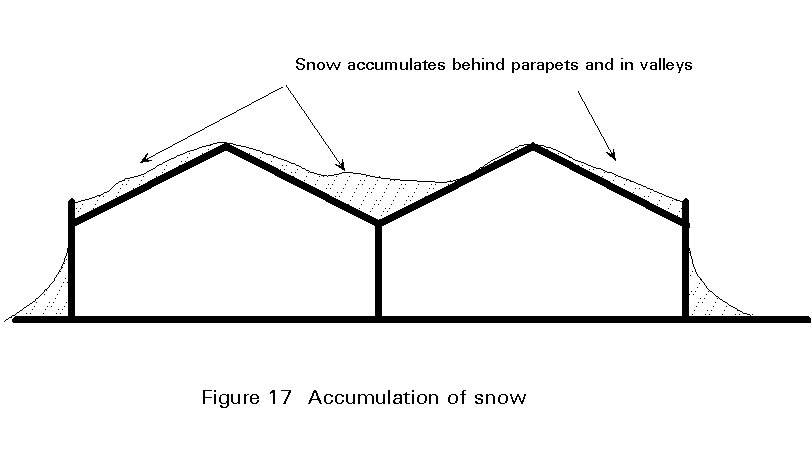
Allowance for different roof configurations can be dealt with by means of a shape coefficient. It provides for conditions such as accumulations of snow behind parapets, in valleys and at abrupt changes of roof height (Figure 17). In addition to snow falling in calm conditions, it may be necessary to consider the effects of wind. Wind may cause a redistribution of snow, and in some cases its partial removal from roofs. Any changes in snow distribution on roofs due to excessive heat loss through part of the roof or snow clearing operations should be accounted for if such loading patterns are critical. Eurocode 1 [1] does not cover additional wind loads due to the presence of snow or the accretion of ice, nor loads in areas where snow is present throughout the year.
For offshore structures in deep and hostile waters, wave loads can be particularly severe. The loads arise due to movement of water associated with wave action. These movements can be described mathematically to relate forces to physical wave characteristics such as height and wavelength.
The treatment is therefore similar to wind loads in that these physical characteristics are predicted and corresponding forces on the particular structural arrangement then calculated. These calculation procedures are, however, very complicated and must realistically be performed on a computer.
Exposed structures such as bridges may be subject to significant temperature variation which must be taken into account in the design. If it is not provided for in terms of allowing for expansion, significant forces may develop and must be included in the design calculations. In addition, differential temperatures, e.g. between the concrete deck and steel girders of a composite bridge, can induce a stress distribution which must be considered by the designer.
Structures for retaining and containing material (granular or liquid) will be subject to a lateral pressure. For liquids it is simply the hydrostatic pressure. For granular material a similar approach can be adopted, but with a reduction in pressure depending on the ability of the material to maintain a stable slope - this is the Rankine approach. Ponding of water on flat roofs should be avoided by ensuring adequate falls (1:60 or more) to gutters.
In some parts of the world earthquakes are a very important design consideration. Seismic actions on structures are due to strong ground motion. They are a function of the ground motion itself and of the dynamic characteristics of the structure.
Strong ground motion can be measured by one of its parameters, the maximum ground acceleration being the parameter most usually adopted for engineering purposes. These parameters are expressed on a probabilistic basis, i.e. they are associated with a certain probability of occurrence or to a return period, in conjunction with the life period of the structure [3].
Accidental actions may occur as a result of accidental situations. The situations include fire, impact or explosion. It is very difficult to quantify these effects. In many cases it may be preferable to avoid the problem, for instance by providing crash barriers to avoid collision from vehicles or roof vents to dissipate pressures from explosions.
Where structures such as crash barriers for vehicles and crowds must be designed for 'impact' the loading is treated as an equivalent static load.
[1] Eurocode 1: Basis of Design and Actions on Structures, CEN (in preparation).
[2] Eurocode 3: Design of Steel Structures: ENV 1993-1-1: Part 1.1, General principles and rules for buildings, CEN, 1992.
[3] Eurocode 8: Structures in Seismic Regions - Design, CEN (in preparation).
[4] Floor Loadings in Office Buildings - the Results of a Survey, BRE Current Paper 3/71, Building Research Establishment, Watford, 1971.
[5] Design Practice and Snow Loading - Lessons from a Roof Collapse, The Structural Engineer, Vol 64A, No 3, 1986.
Part 1 Butterworths, 1985
Part 2, Butterworths, 1990.
|
Loaded Areas |
a [kN/m2] |
|
|
Category A
Category B
Category C
Category D |
- general - stairs - balconies
- general - stairs, balconies
- with fixed seats - other
- general |
2,0 3,0 4,0
3,0 4,0
4,0 5,0
5,0 |
Table 1 Imposed loads on floors in buildings
|
|
Snow load so [kN/m2] |
|||
|
Altitude [m] |
||||
| Zone |
0 |
200 |
400 |
600 |
|
1 |
0,40 |
0,49 |
0,70 |
0,95 |
|
2 |
0,80 |
0,98 |
1,40 |
1,89 |
|
3 |
1,20 |
1,47 |
2,09 |
2,84 |
|
4 |
1,60 |
1,97 |
2,79 |
3,78 |
|
5 |
2,00 |
2,46 |
2,49 |
4,73 |
Table 2 Snow loads for zones given in Figure 16
so = 0,412z ![]()
where:
A is the altitude of the site above mean sea level [m]
z is a constant, depending on the snow load zone.